17 Branches of Mathematics and Their Definitions
Mathematics is a formal science in which we often use letters, symbols, and signs. it is derived from the Greek mathema, which can be translated as “study of a subject. ” it also makes us able to solve problems. Arithmetic, Algebra, Analysis of Functions, Geometry, Statistics, and Probability are some important Branches of Mathematics.
before going to dig deeper into branches of mathematics, let’s have a bit of an introduction to mathematics.
What is mathematics?
Before we dig deeper into branches of mathematics (the queen of sciences) let’s have a brief introduction to mathematics.
So, According to www.mariam-webster.com, Mathematics is the science of numbers and their operations, interrelations, combinations, generalizations, and abstractions and of space configurations and their structure, measurement, transformations, and generalizations. (source)
What are the main branches of mathematics?
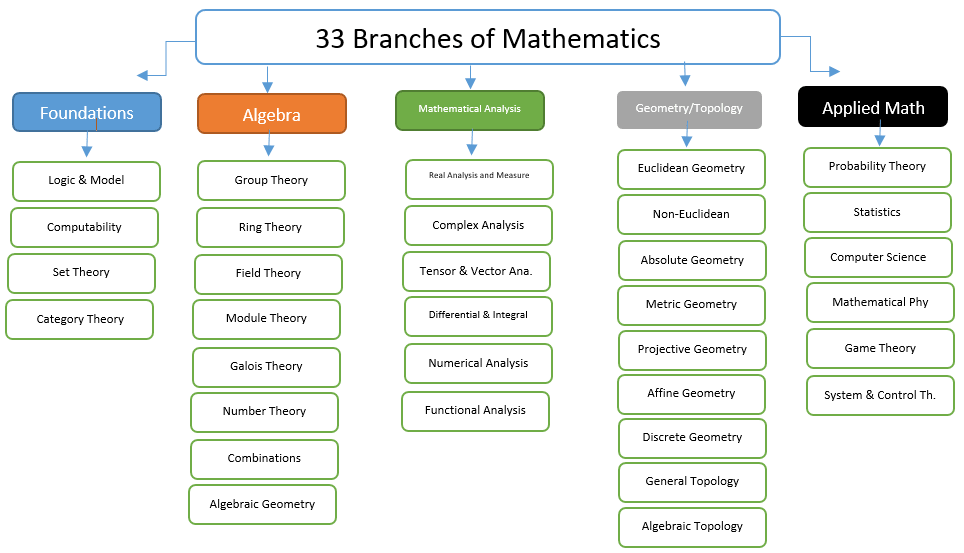
with the immense & rapid progress in science, there is a variety of branches and sub-branches found in mathematics. however, there are 16 major branches/divisions which are given below:
- Geometry
- Algebra
- Arithmetic
- Trigonometry
- Infinitesimal calculus
- Mathematical analysis
- Mathematical logic
- Game theory
- Discrete mathematics
- Graph theory
- Number theory
- Topology
- Pure mathematics
- Applied mathematics
- Combinatorics
- Experimental mathematics
- Order theory or Set Theory
here’s the detail of each branch along with their sub-branches.
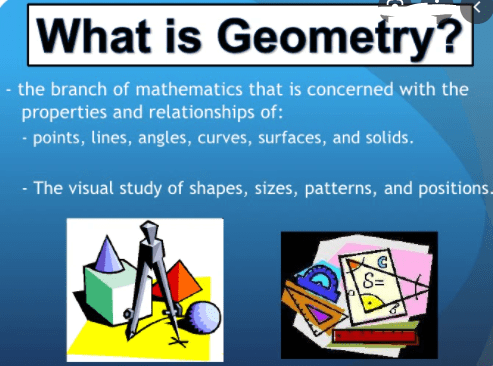
Geometry:
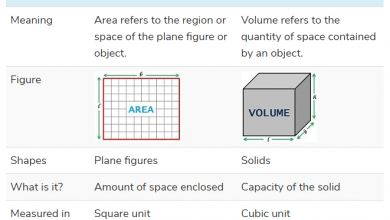
Geometry is another important branch of mathematics that deals with the study of figures in space and their links in different dimensions, generally two or three. It also studies the relationships between points, lines, lines, and other figures.
Geometry can be divided into two further branches such as trigonometry and descriptive geometry. it helps to solve triangles and complex numbers. we often discuss many other things in trigonometry like Vectors, planes, lines, etc.
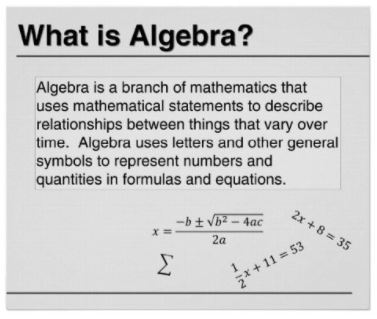
Algebra:
It is a branch of mathematics that is dedicated to analyzing different structures and studies a combination of elements, Operations with polynomials, equations, inequalities, and systems of equations. it also relates to performing arithmetic operations through letters or symbols, Matrices, determinants, and linear programming.
Arithmetic:
Arithematics focuses on understanding and study of numbers and their different operations with integers, rational, irrational, real, and complex numbers Powers, radicals, and logarithms, etc. furthermore, it deals with the elementary operations of addition, subtraction, division, and multiplication. it is considered the basic branch of mathematics from which other branches spread out.
Infinitesimal calculus:
The infinitesimal calculus or calculus of infinitesimals constitutes a very important part of modern mathematics. It’s normal, just call it calculus. Calculus, as an algorithm developed in the field of mathematics, includes the study of limits, derivatives, integrals, and infinite series. Specifically, infinitesimal calculus is the study of change, in the same way, that geometry is the study of space.
The infinitesimal calculus is a first-rate scientific and technological tool: undoubtedly the most powerful and efficient for the study of nature that mathematicians have ever developed. What makes it so versatile is the great variety of processes of all kinds, mathematical, physical, technological, economic, and biological. which are modeled and solved using infinitesimal calculus.
Paraphrasing Galileo, it can be said that infinitesimal calculus is the language of nature. However, few people know the great versatility of the basic concepts of calculus: the derivative and the integral.
The infinitesimal calculus or calculus of infinitesimals constitutes a very important part of mathematics that studies concepts such as functions, limits, derivatives, integrals, and infinite series. It is very common in the academic context, for convenience, simply call it calculus.
More specifically, infinitesimal calculus is the study of change, in the same way, that geometry is the study of space. Infinitesimal calculus has wide applications in science and engineering and allows us to solve those problems for which algebra alone is insufficient.
Infinitesimal calculus includes two main fields, differential calculus, and integral calculus, which are related to the fundamental theorem of calculus. In more advanced mathematics, calculus is usually called mathematical analysis, or simply analysis, and is defined as the study of functions.
Mathematical analysis:
Mathematical analysis is a branch of mathematics. This focuses on the study of real and complex numbers, as well as their representation; even using letters.
Mathematical analysis, in particular, addresses topics such as derivatives, integrals, limits, series, and various types of complex functions. The purpose of mathematical analysis is to solve complex calculations through abstraction. To do this, it uses tools such as functions.
Mathematical analysis is an area of mathematics that studies the behavior of real numbers (which are the basis on which part of the analysis in mathematics ) and complex numbers.
It also covers the constructions obtained from these numbers, such as functions, series, sequences, continuity, limits, and convergence, as well as the branches of integration and derivability.
Mathematical logic:
Mathematical logic analyzes certain proposition seeking to identify whether it represents a true or false statement.
At first, logic was linked to philosophy, having been initiated by Aristotle (384-322 BC) who was based on the theory of the syllogism, that is, on valid arguments.
In mathematical logic, we use propositional calculus, which resembles traditional mathematical calculus
As with mathematical calculations, in logic, there are some symbols and concepts that are fundamental for understanding and solving exercises. The “reasoning” we use can be classified as propositional calculus. An initial idea of logic is to classify something as true or false. Suppose a friend told you that “a pear is a fruit”.
Game theory:
Game theory is a branch of economics that studies the decisions in which for an individual to be successful he has to take into account the decisions made by the rest of the agents that intervene in the situation. Game theory as a mathematical study has not been used exclusively in economics, but in management, strategy, psychology, or even biology.
Game theory is a branch of mathematics and economics that studies the choice of the optimal behavior of an individual when the costs and benefits of each option are not fixed in advance but depend on the choices of other individuals.
Discrete mathematics:
Discrete mathematics corresponds to an area of mathematics that is responsible for studying the set of natural numbers; that is, the set of countable finite and infinite numbers where the elements can be counted separately, one by one.
These sets are known as discrete sets; An example of these sets are integers, graphs, or logical expressions, and they are applied in different fields of science, mainly in computer science or computing.
Graph theory:
Graph theory is an extraordinary mix of history, culture, and solutions to complex problems from the world of mathematics. This theory seeks to visually represent sets of abstract data in form of nodes or vertices and the union or relationships that these can have with other nodes through edges. Thanks to this theory, great advances have been made in the analysis of large volumes of data.
Today graphs are a central part of an incredible number of solutions to complex problems. They are one of the fundamental components of social analytics, and relationships between people, and more recently their properties have been used to increase efficiency in the fight against money laundering and bank fraud.
Number theory:
Number theory is the branch of pure mathematics that studies the properties of numbers in general and integers in particular, as well as various problems derived from their study.
It contains a considerable number of problems that could be understood by “non-mathematicians.” More generally, this field studies the problems that arise with the study of integers.
As Jürgen Neukirch cites: Number theory occupies an idealized position among mathematical disciplines analogous to that occupied by mathematics itself among the other sciences. The arithmetic term was also used to refer to number theory.
This is a fairly old term, although not as popular as in the past. Hence number theory is often called high arithmetic, although the term has also fallen into disuse. This sense of the term arithmetic should not be confused with elementary arithmetic, or with the branch of logic that studies Peano’s arithmetic as a formal system. Mathematicians who study number theory are called number theorists.
Topology:
The term topology is used to identify an area of mathematics that studies continuity and other concepts originating from it. It is a specialization linked to the properties and characteristics that geometric bodies possess and that remain unaltered thanks to continuous changes, regardless of their size or appearance.
It should be noted that the continuous functions of mathematics are those that, in the close points of the domain, experience small variations in the values. At a graphical level, these functions are usually able to be drawn without having to lift the pencil from the paper.
If you have features that coincide and share the same coordinate location, boundaries, or nodes, the geodatabase topology can help you better manage your geographic data.
Geodatabase topologies help ensure data integrity. Using a topology provides a mechanism to perform integrity checks on your data and helps you validate and maintain better representations of the features in your geodatabase.
Additionally, topologies can be used to model spatial relationships between entities. In this way, you can perform various analytical operations, such as finding adjacent entities, using coincident boundaries between entities, and navigating connected entities.
Pure mathematics:
Applied mathematics:
Combinatorics:
Experimental mathematics:
Order theory:
Importance of mathematics in everyday life
You May Also Like: